 |
| The Pythagoras' Theorem |
The Pythagorean theorem is also known as the Pythagoras' theorem. It is named after a famous Greek mathematician, Pythagoras, who is credited for its proof.
The theorem states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the remaining sides. That is c^2 = a^2 + b^2, where c is the hypotenuse, a and b are the lengths of the remaining sides.
Here is one of the theorem. Take note that the theorem only applies to right triangles.
Start with a cutout of any right triangle.
Let us name the shortest side as a, the longer side as b and the hypotenuse as c.
Let us make three (3) more of the right triangle.
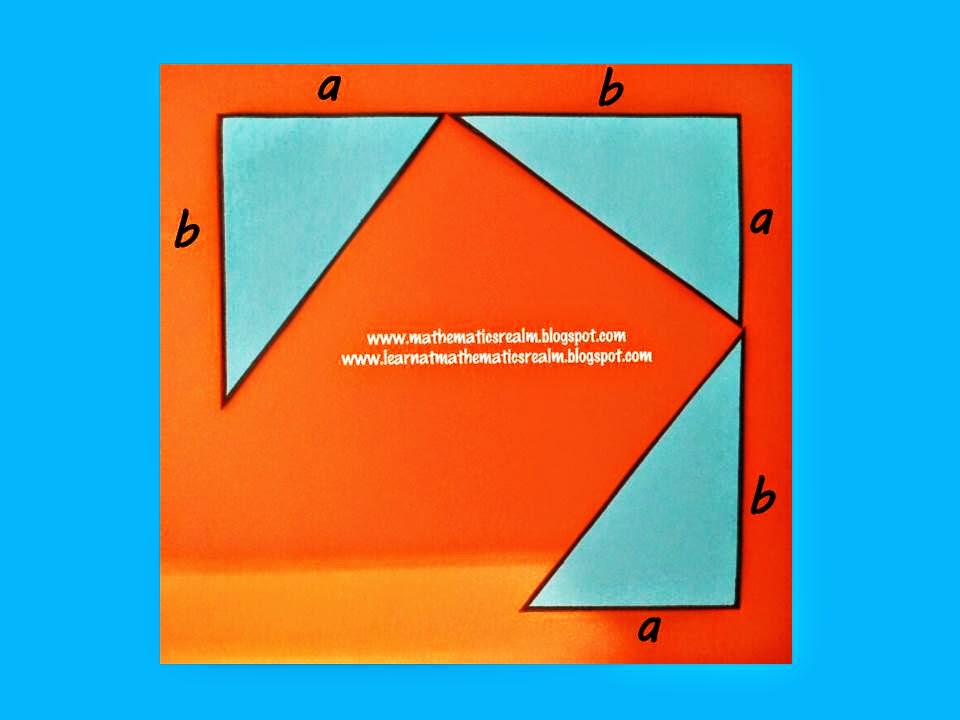
Our next step is to form a square out of the four (4) triangles, making sure that the combined length of a and b will be the length of the side of the square that we are forming.
Let us take a look at the different parts of the square we formed. If we separate the whole square, we can get its area in terms of a and b.
The middle part is also a square with sides equal to the hypotenuse of the right triangle. The area can also be obtained.
The area of the four right triangles outlining the sides of the whole square is as follows
We take note that the area of the whole square is equal to the area of the small square in the middle added to the area of the four right triangles. That is
Thus, c^2 = a^2 + b^2.
Hope this will help you understand the derivation of the Pythagoras' Theorem.
Your comments and suggestions are welcome here. You may write them in the comment box below. Thank you!
The Pythagorean theorem, attributed to the ancient Greek mathematician Pythagoras, is a cornerstone of geometry that fundamentally relates the sides of a right triangle. It elegantly states that the square of the hypotenuse (c) is equal to the sum of the squares of the other two sides (a and b). This theorem not only serves as a critical tool in mathematics but also exemplifies the beauty of mathematical proofs and their application in real-world scenarios. Its simplicity and profound implications highlight the enduring legacy of Pythagoras and the timeless nature of mathematical discovery.
ReplyDeleteThis is a very clear and practical way to explore the Pythagorean Theorem. I especially like how the four right triangles are arranged to form a larger square and how the area of the inner square leads to the relationship between the three sides. Visual demonstrations like this make the theorem much easier for students to understand rather than simply memorizing the formula. For anyone practicing right-triangle calculations, a Pythagorean Theorem Calculator can also be useful for checking different examples: https://pythagorastheoremcalculation.co/
ReplyDeleteI really like this visual approach to exploring the Pythagorean Theorem. Rearranging the four right triangles to create the larger square makes the relationship between the areas much easier to understand than simply starting with a² + b² = c². It would be a great classroom activity because students can actually see why the theorem works instead of only memorizing the formula. I’ve also been exploring practical ways to check right-triangle calculations here: https://pythagorastheoremcalculation.co/
ReplyDelete