Archive for 2013
THE SUM OF THE ANGLES OF A TRIANGLE (Part 2: Exploration)

This is the second part of the proof that the sum of the measures of the angles of a triangle is 180 degrees. This is actually the second method. The first one is more on using manipulatives or visual representations. This time, let us use basic mathematical concepts in proving.
This method is applicable to any type of triangle.
Let us use only one of the triangles. The process will be the same for the other triangles. To start with, let us name the triangle as ABC.
Now, let us draw a line parallel to base AC passing through B. Let us name this line as line BD or line BE or line ED, in any way you want it.
Since the focus of this proof is on the angles, let us rename each angle using numbers. It will be easier for us to determine the angles using the numbers instead of using three letters.
In this case,
Since we have drawn a line parallel to line AC, then we could say that side AB and side side BC are transversals of the parallel lines BD and line AC. Let us recall the concept of alternating interior angles for parallel lines.
Since we know that these alternate interior angles are always equal, then in the figure that we have formed, angle 1 = angle 4 and angle 3 = angle 5.
If you notice, angles 2, 4 and 5 form a straight line. Let us recall
That means the sum of angles 4, 2 and 5 is 180 degrees, because they form a straight line.
From the illustrations above, let us recall that angle 1 = angle 4 and angle 3 = angle 5.
Further, it means that the sum of angles 1, 2 and 3 is also 180 degrees.
Therefore, we can conclude that
You can also use the other sides of the triangle for the proof. The same process will be used in each of the cases.
Here is the summary of the proof in pdf form. You may download and print for academic use. Hope it will become useful to you.
You comments ad suggestions are welcome here. Write them down in the comment box below. Thank you!
THE SUM OF THE ANGLES OF A TRIANGLE (Part 1: Exploration)

Everybody knows that the sum of the measures of the angles of any triangle is 180 degrees. If I may ask each one of you why, one of the reasons that I may probably hear is that "Our math teacher told us!" - which should not be the case. You should know how to show that the sum of the angles of any triangle is really 180 degrees. Where do 180 degrees come from? Why 180 degrees? Why not 360 degrees?
The purpose of this post is to show you how to prove that the sum of the angles of any triangle is really 180 degrees. This is the first method, which is the elementary way - the easiest way, to prove it. The other methods will also be posted here.
PROVE: The sum of the measures of the angles of any triangle is 180 degrees.
PRE-REQUISITE: The sum of the measures of the angles that form a straight line is 180 degrees.
MATERIALS NEEDED: colored papers or cardboards, pair of scissors, ruler, marker
PROCEDURES:
1. Cut three different types of triangles, classified according to angles. Use the colored papers or cardboards for the triangles. One should be a right triangle, another should be an acute triangle and the last should be an obtuse triangle.
2. For us to easily identify the angles of the triangles later, highlight the edges of each triangle using a black (or any dark colored) marker.

3. Using a pair of scissors or cutter, cut the sector/region of the angles of each triangle.
4. For each of the triangles, arrange the regions of the angles in such a way that they are adjacent to each other. Notice that in this case, the lower part of the angles form a straight line.
5. In each of the figures formed, the angles formed a straight line at the bottom part. Recall that the sum of the angles that form a straight line is 180 degrees.
CONCLUSION: The sum of the measures of the angles of any triangle is 180 degrees.
For educators and parents, I have made a worksheet for this activity for your class/children. You are free to download and print it. You may group your students and let each group work on a triangle or all the triangles. I hope this will be helpful.
1 = 2?
Maybe you are wondering why or how can 1 be equal to 2. Let us look at the following proof:
If we simplify both sides by combining like terms, it will become
Now let us subtract 2a on both sides
The right side has a common factor, which is 2. Using distributive property, it can be rewritten as
If you notice, both sides has a common factor, which is b - a. To simplify the equation, let us divide both sides by the common factor.
The process will arrive at
Are you convinced? No no no...
Seems like the proof is valid but there is something wrong with it. Look over the proof once again. Can you identify which of the process is not valid?
There is nothing wrong with the given. Real numbers can be equal. There is nothing wrong also with adding b and subtracting 2a on both sides. Likewise, there is nothing wrong with combining like terms on both sides. Then, where is the mistake?
There is nothing wrong with dividing both sides by any number but in this case it becomes invalid. The reason is that b - a = 0 since a = b. Subtracting equal numbers will yield 0. Since b - a = 0, then the result will be UNDEFINED. We cannot also cancel out b - a on both sides because of that.
Therefore, 1 is not equal to 2.
Here is a copy of the proof in pdf form. You may download and print it for educational purposes. You may share it to your friends. Your comments and suggestions are also welcome here.
Sunday, April 14, 2013
GRAPHING PAPER (SIZE A4) FOR DOWNLOAD
This is the second version of the downloadable graphing paper that can be used for math and science classes. The size of this grid paper is a4 (8.27in. x 11.69 in or 210mm x 297 mm). You may directly print it also.
You are also free to write your comments and suggestions about this below (using the comment box). Thank you!
Thursday, April 4, 2013
GRAPHING PAPER (LETTER SIZE) FOR DOWNLOAD
This is a graphing paper in letter size (8.5 in. x 11 in.). You can download this pdf file using the download arrow below. You can directly print it also.
I hope this may help you in any way. If there are any suggestions or comments about this graphing paper, please inform me through the comment box below. Thank you!
HELLO WORLD!
Welcome to a new site of Mathematics Realm. This is an extension of the realm where you can find discussions, tutorials, and downloads for the different branches of mathematics.
It will include basic to complex topics in mathematics. First few topics will focus on the basic concepts. This is to ensure clarity and understanding on the foundations.
Join me as we start our journey with mathematics. I hope eventually you will learn how to love mathematics.
Comments will be accepted from readers especially if there are corrections or suggestions. I would be glad to hear them from you.
<a href="http://www.bloglovin.com/blog/6552029/?claim=y7sgveja96j">Follow my blog with Bloglovin</a>































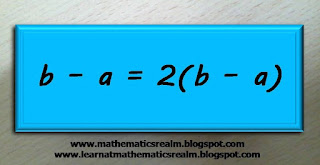





.jpg)
.jpg)