Visual and Geometric Proof of Algebraic Identities
There are a lot of algebraic identities. One of the most commonly used identity is the identity
(a+b)^2 = a^2 + 2ab + b^2.
Did you ever wondered where did it came from?
There are various ways on how to prove this algebraic identity. One of which is using the visual and geometric way. It used basic concepts such as areas of squares and rectangles to derive the identity. In the following video, the prerequisite concepts are also included to support the derivation process. The visual proof or geometric representation is shown in detailed and in step-by-step manner. This is for you to easily understand the concept. You may watch the following video:
The same algebraic identity can also be proven using basic algebraic processes. Some of the prerequisite concepts included are multiplication law of indices and distributive property of multiplication. These prerequisites help in the derivation process of the algebraic identity (a+b)^2 = a^2 + 2ab + b^2. You may watch the complete details in this video:
Further, the algebraic identity can also be used as a guide in expanding the square of any binomials. An acronym S-2P-S is introduced in the following video for you to easily remember the process of expanding the square of binomials the fastest way. Here is the complete discussion of the acronym with various examples:
Hope you will learn from these videos about algebraic identities.
Your comments and suggestions are welcome here. Write them in the comment box below. Thank you and God bless!
------------------------------------------------------------------------------------------------------------- Thank you for your continued support!
Please subscribe and share!
Youtube Channel: CIE Math Solutions
Instagram: @ciemathsolutions
Twitter: @ciemathsolution
Facebook Page: CIE Math Solutions
Monday, September 28, 2020
PYTHAGOREAN THEOREM (Proof by Rearrangement: Part 1)
Here is another proof of the Pythagorean theorem.
Let us use a right triangle and name the shortest side as a, the longer side as b, and the hypotenuse as c.
Let us make three more of these so we have four congruent right triangles.
Now, let us arrange the four right triangles to form a square like this
In this figure, there are two squares formed. The first square is larger square, with side equal to a+b, while the second square is the inner square with side equal to c.
Let us focus on the inner square. The length of its side is equal to the hypotenuse of the four right triangles. It means that it has sides each measuring as c. Hence, the area of the square is c^2.
Let us take note of that the ares of the inner square is c^2.
Now, let us label the four right triangles as triangle 1, triangle 2, triangle 3 and triangle 4. This will make it easier for us to identify which triangle is moved later.
Let us rearrange the triangles. Let us move triangle 2 beside triangle 1, and triangle 4 beside triangle 3. In this case, each pair will form a rectangle.
Let us focus on the area being left by the two triangles and shade it with white.
If you notice, the white area can be divided into two like this
We formed two quadrilaterals but we are not yet sure if they are squares or not.
The smaller quadrilateral has a side that is equal to the shortest side of triangle 4. This means that this side measures a.
On the other hand, if we slide back triangle 2, we could see that the upper side of the small quadrilateral is also the shortest side of triangle 2.
This means that the upper side of the small quadrilateral measures a. Hence, the small quadrilateral is a square.
The area of the small square is
Now, let us look at the bigger quadrilateral shaded with white. One of its side (leftmost) has the same length as the longer side of triangle 2. It means that this side measures b.
On the other hand, if we slide back triangle 4, we could see that its longer side coincides with the lower side of the big white quadrilateral.
This means that the measure of the lower side of the big white quadrilateral is b. Hence, the big white quadrilateral is a square.
The area of the big white square is
If we compare the two figures formed. Both of them has four (4) right triangles and the areas of these triangles are the same. It means that the area of the white inner square in the first figure is the same as the area of the two white squares in the second figure.
Therefore, for any right triangles
Let us use a right triangle and name the shortest side as a, the longer side as b, and the hypotenuse as c.
Let us make three more of these so we have four congruent right triangles.
Now, let us arrange the four right triangles to form a square like this
In this figure, there are two squares formed. The first square is larger square, with side equal to a+b, while the second square is the inner square with side equal to c.
Let us focus on the inner square. The length of its side is equal to the hypotenuse of the four right triangles. It means that it has sides each measuring as c. Hence, the area of the square is c^2.
Let us take note of that the ares of the inner square is c^2.
Now, let us label the four right triangles as triangle 1, triangle 2, triangle 3 and triangle 4. This will make it easier for us to identify which triangle is moved later.
Let us rearrange the triangles. Let us move triangle 2 beside triangle 1, and triangle 4 beside triangle 3. In this case, each pair will form a rectangle.
Let us focus on the area being left by the two triangles and shade it with white.
If you notice, the white area can be divided into two like this
We formed two quadrilaterals but we are not yet sure if they are squares or not.
The smaller quadrilateral has a side that is equal to the shortest side of triangle 4. This means that this side measures a.
On the other hand, if we slide back triangle 2, we could see that the upper side of the small quadrilateral is also the shortest side of triangle 2.
This means that the upper side of the small quadrilateral measures a. Hence, the small quadrilateral is a square.
The area of the small square is
Now, let us look at the bigger quadrilateral shaded with white. One of its side (leftmost) has the same length as the longer side of triangle 2. It means that this side measures b.
On the other hand, if we slide back triangle 4, we could see that its longer side coincides with the lower side of the big white quadrilateral.
This means that the measure of the lower side of the big white quadrilateral is b. Hence, the big white quadrilateral is a square.
The area of the big white square is
If we compare the two figures formed. Both of them has four (4) right triangles and the areas of these triangles are the same. It means that the area of the white inner square in the first figure is the same as the area of the two white squares in the second figure.
Therefore, for any right triangles
Your comments and suggestions are welcome here. Write them in the comment box below.
Don't forget to like and share.... :)
Thank you and God bless!
Don't forget to like and share.... :)
Thank you and God bless!
Sunday, January 25, 2015
PYTHAGOREAN THEOREM EXPLORATION 2 (CUT-OUTS)
 |
| Pythagorean Theorem Exploration 2 Cutouts |
You may download this template for personal use and for your math class activity. The length of the sides on the second page measures 6.5 inches. This is also the measure of the length of the hypotenuse of the right triangle on the first page.
Your comments and suggestions are welcome here. Write them in the comment box below.
Don't forget to like and share.... :)
Thank you and God bless!
Don't forget to like and share.... :)
Thank you and God bless!
Friday, November 21, 2014
PYTHAGOREAN THEOREM EXPLORATION 1 (CUT-OUTS)
 |
| Pythagoras Theorem Cutouts |
The Pythagoras Theorem states that:
"The square of the length of the hypotenuse (denoted by c) is equal to the sum of the squares of the lengths of the other two sides (denoted by a and b)"
 |
| Pythagoras Theorem |
Here is the cutout for the first exploration of the Pythagoras Theorem. The file consist of two pages. The first page consists of four congruent right triangles. The second page is a square.
Cut along the broken lines on the right triangles and use them to fill in the square on the second page using the EXPLORATION 1 as a guide.
You comments and suggestions are welcome here. You may write them in the comment box below.
Monday, November 17, 2014
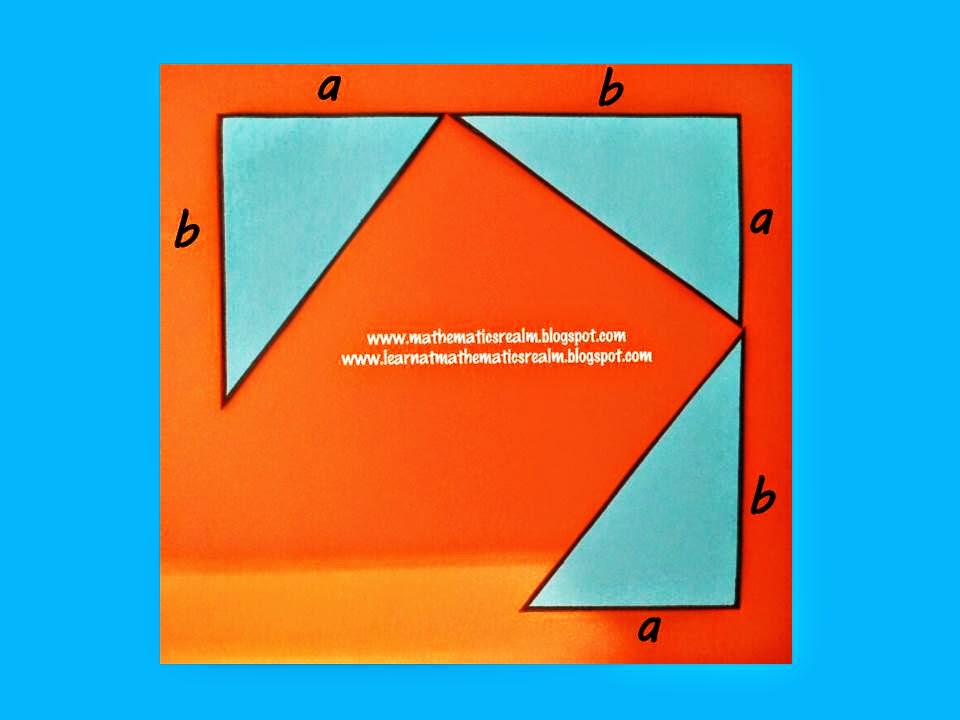
PYTHAGOREAN THEOREM (Exploration 2)
 |
| The Pythagorean Theorem |
Start with a cutout of a right triangle.
Cut three more cutouts.
Let us name the sides of the right triangle with c as the hypotenuse.
Now, let us form a square using the four (4) triangles. This time, use the hypotenuse as the sides of the square.
Let us take a look at the different parts of the square we formed. If we separate the whole square, we can get its area in terms of c.
The middle part is also a square with sides equal to the difference of sides b and a. The area can be obtained as
On the other hand, the area of the four triangles outlining the sides of the whole square is as follows.
Combining their areas, we obtain
Thus, c^2 = a^2 + b^2.
Your questions, comments and suggestions are welcome here. Kindly write them in the comment box below.
Sunday, August 17, 2014
PYTHAGOREAN THEOREM (Exploration 1)
 |
| The Pythagoras' Theorem |
The theorem states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the remaining sides. That is c^2 = a^2 + b^2, where c is the hypotenuse, a and b are the lengths of the remaining sides.
Here is one of the theorem. Take note that the theorem only applies to right triangles.
Start with a cutout of any right triangle.
Let us name the shortest side as a, the longer side as b and the hypotenuse as c.
Let us make three (3) more of the right triangle.
Our next step is to form a square out of the four (4) triangles, making sure that the combined length of a and b will be the length of the side of the square that we are forming.
Let us take a look at the different parts of the square we formed. If we separate the whole square, we can get its area in terms of a and b.
The middle part is also a square with sides equal to the hypotenuse of the right triangle. The area can also be obtained.
The area of the four right triangles outlining the sides of the whole square is as follows
We take note that the area of the whole square is equal to the area of the small square in the middle added to the area of the four right triangles. That is
Thus, c^2 = a^2 + b^2.
Hope this will help you understand the derivation of the Pythagoras' Theorem.
Your comments and suggestions are welcome here. You may write them in the comment box below. Thank you!
Saturday, May 24, 2014



















.jpg)